Professor Chaim Goodman-Strauss of the Department of Mathematical Sciences is part of an interdisciplinary team that recently announced a solution to the "einstein" or "one stone" problem, a tantalizing question that has been asked for more than 50 years.

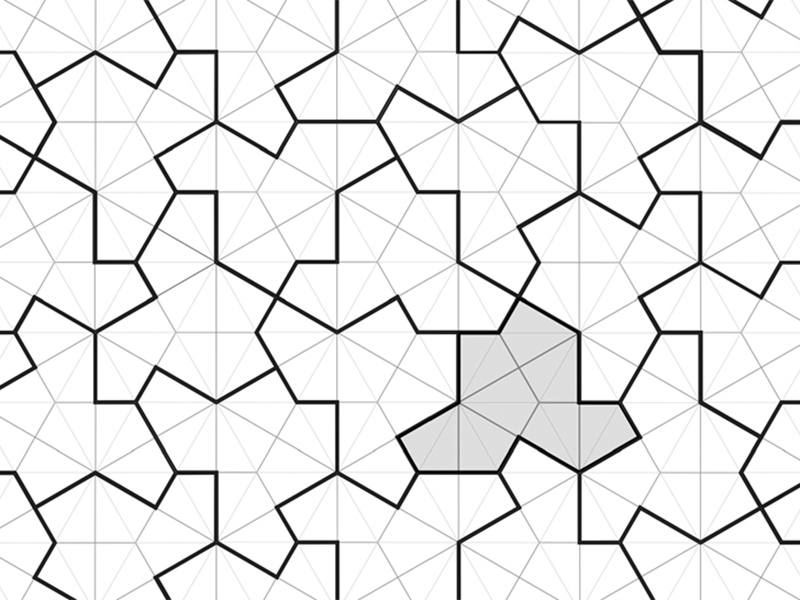
This problem asks about the ways in which a plane can be tiled. Intuitively, a tiling consists of breaking the plane into pieces without gaps or overlaps. Examples of tilings abound in the real world and in nature.

For example, an infinite checkerboard gives a tiling of the plane by squares. Other tilings are visible in the hexagons of a honeycomb or the tile mosaics of the Alhambra. These examples of tilings are periodic, though, that is they possess a translational symmetry. We can imagine picking up the infinite checkerboard, sliding it up one square and then placing it back down where each piece still fits exactly into the checkerboard pattern.

Surprisingly, there exist finite collections of shapes that do tile the plane, but where none of their tilings have a translational symmetry. These are called aperiodic tile sets. The first examples were created in the 1960s and needed over 20,000 different shapes. This number was slowly reduced, and in the 1970s the British mathematician Sir Roger Penrose demonstrated an aperiodic tile set that used just two shapes. The question remained, is there an aperiodic tile set with a single shape?

Such a shape was just found by this interdisciplinary research team including professor Goodman-Strauss. The proof that this shape is indeed an aperiodic tile set appears a new preprint. This announcement generated a lot of excitement in and beyond the worlds of mathematics and computer science and was featured in a recent New York Times article.

"This is something I did not think I would see in my lifetime," said professor Edmund Harriss of the Department of Mathematical Sciences, "and it is beautiful that it was such an interdisciplinary effort. You have David Smith, a retired printing technician who had been seriously exploring ways to tile the plane for many years, who created the shape, and Joseph Myers, a software developer who found the two proofs, working together with Craig Kaplan, a computer science professor at the University of Waterloo, and Chaim Goodman-Strauss."